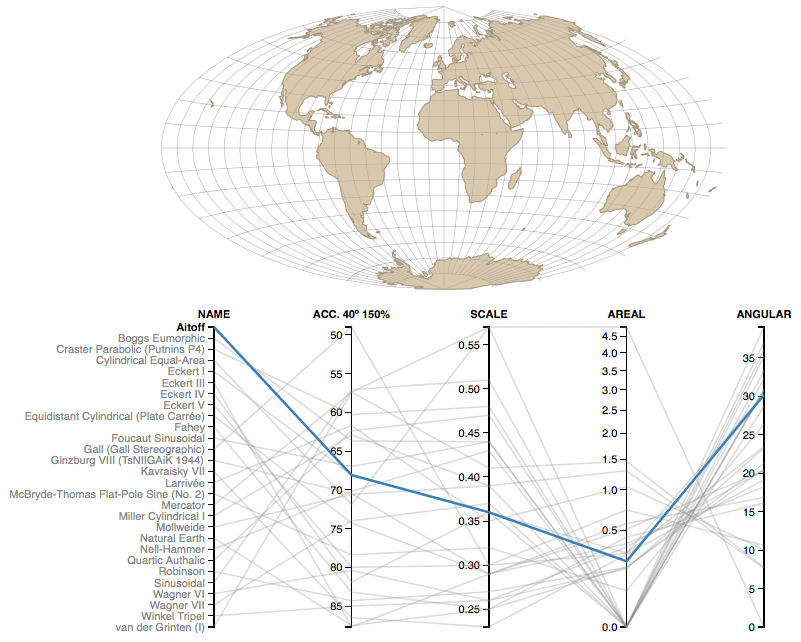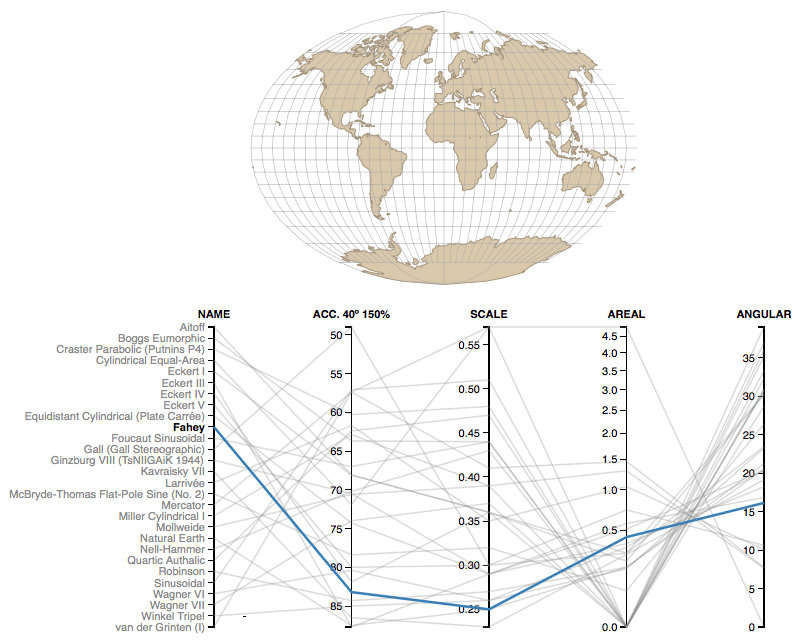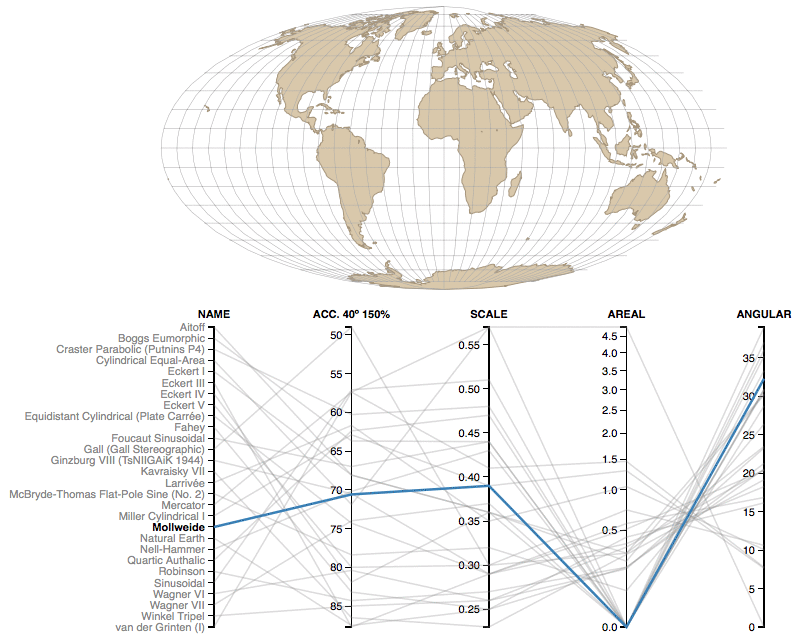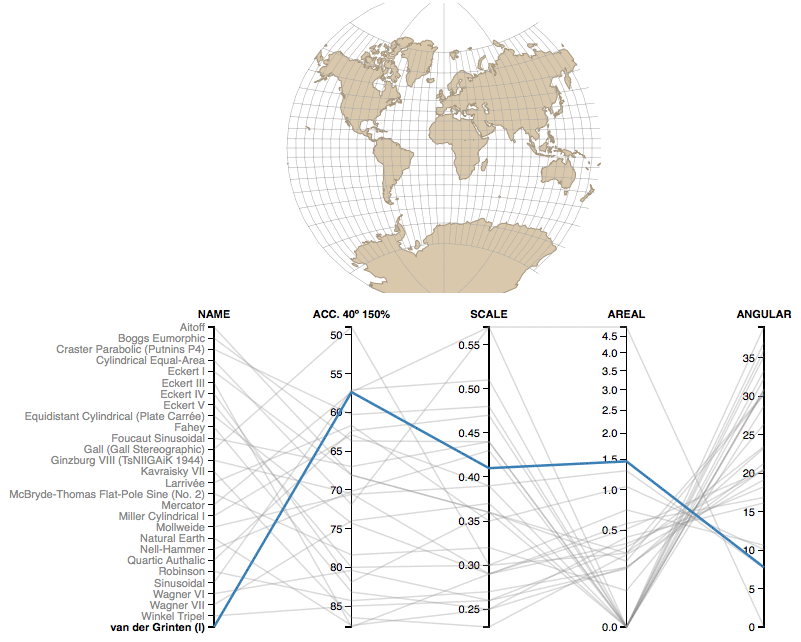
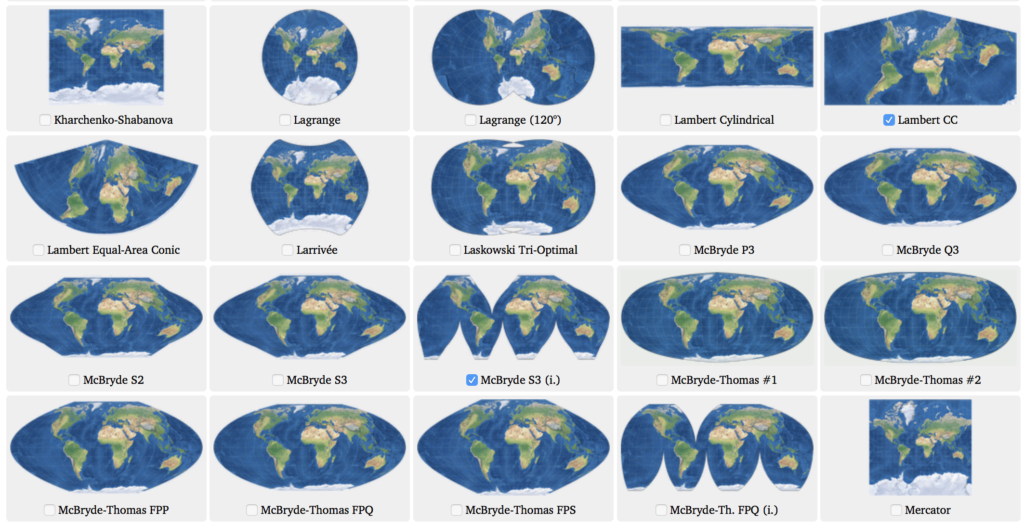
#Ideas
Amazing visualization of how Christianity spread around the world
From a sect of Jews to a world dominating religion with over 2 billion followers, the story of Christianity is fascinating. This animated map gives a geographic look at the past 2,000 years and shows how the religion was spread around the world, first by Jesus’s disciples, then by emperors, kings, and missionaries.
It is also interesting to see how all five of the biggest religions in the world: Hinduism, Buddhism, Christianity, Judaism, and Islam spread across our planet.
The video is a part of a larger Business Insider series. You can find all of these videos here.

#Ideas
#Contributing Writers
#GeoDev
#Ideas
Must-Have QGIS Plugins To Elevate Your Mapping Projects
#GeoAI
#Ideas
#Insights
The Deep Tech Revolution in Geospatial: Where Science Meets Real-World Impact
#GeoDev
#Ideas
#Insights
Explaining 3D Tiles: The New Standard for Scalable Geospatial Data